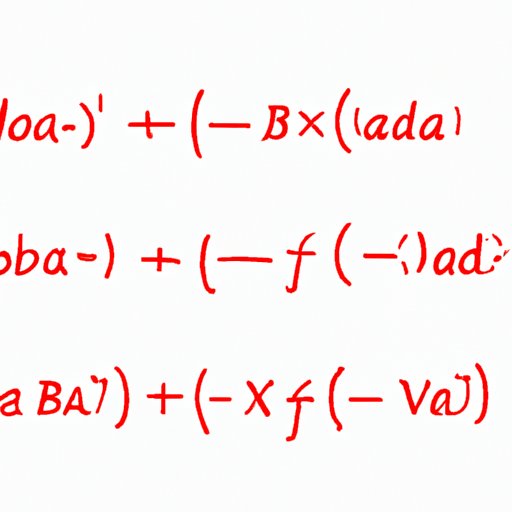Introduction
Quadratic equations may seem intimidating but are an important concept in mathematics and are used in many real-life applications such as science and engineering. However, solving quadratic equations can be challenging for students due to their complexity. In this article, we will take a practical, step-by-step approach to solving quadratic equations, using simple examples, and avoiding common mistakes.
Mastering the Basics: A Beginner’s Guide to Solving Quadratic Equations
Before diving into solving quadratic equations, we need to define and understand the key terms. The quadratic equation is an equation of the second degree, which means it has the highest exponent of two. A variable is a symbol or a letter used to represent an unknown number in an equation, such as x or y. A coefficient is the number that appears before a variable, such as 2x, where 2 is the coefficient of x.
The quadratic formula is the formula used to solve quadratic equations, and it is given by:
x = (-b ±√(b²-4ac))/(2a)
where a, b, and c are coefficients of the quadratic equation in the form ax² + bx + c = 0.
Let’s take the example of a quadratic equation, 2x² + 5x + 3 = 0, and understand how to use the quadratic formula. We simply substitute a=2, b=5, and c=3 in the formula.
x = (-5 ±√(5²-4*2*3))/(2*2)
x = (-5±√1)/4
Simplifying, we get two solutions for x: -3/2 and -1. To verify, we can substitute these values into the original quadratic equation and see if it equals 0.
Breaking It Down: Step-By-Step Instructions for Solving Quadratic Equations
Now, let’s walk through the quadratic equation-solving process step-by-step and offer examples of problems at varying levels of difficulty. We will cover different methods of solving quadratic equations, including factoring and completing the square.
In general, there are three methods for solving quadratic equations:
- Factoring
- Completing the square
- Using the quadratic formula
Factoring is the process of breaking down the quadratic equation into two binomial factors whose product equals the original quadratic equation. Completing the square is a process where we add and subtract a constant to the quadratic equation so that we can write it as the square of a binomial.
Let’s take the example of quadratic equations, x² + 5x + 6 = 0, and x² – 2x – 3 = 0, and solve them using all three methods.
Example 1: x² + 5x + 6 = 0
Factoring: To factor this equation, we need to find two numbers whose product equals 6 and whose sum equals 5. The two numbers are 2 and 3. Therefore, we can write x² + 5x + 6 = (x+2)(x+3) = 0. Solving for x, we get x=-2 and x=-3.
Completing the square: To complete the square, we need to add and subtract a constant to the quadratic equation. First, we take the coefficient of x, which is 5, and divide it by 2. We get 2.5. Next, we square 2.5 and get 6.25. We can now add and subtract 6.25 from the quadratic equation to get:
x² + 5x + 6 = (x+2.5)² – 0.25 = 0
Solving for x, we get x=-2 and x=-3.
Quadratic formula: We already know the quadratic formula.
Substituting our values, we get:
x = (-5 ±√(5²-4*1*6))/(2*1) = -2, -3
Example 2: x² – 2x – 3 = 0
Factoring: To factor this equation, we need to find two numbers whose product equals -3 and whose sum equals -2. The two numbers are -3 and 1. Therefore, we can write x² – 2x – 3 = (x-3)(x+1) = 0. Solving for x, we get x=3 and x=-1.
Completing the square: To complete the square, we need to add and subtract a constant to the quadratic equation. First, we take the coefficient of x, which is -2, and divide it by 2. We get -1. Next, we square -1 and get 1. We can now add and subtract 1 from the quadratic equation to get:
x² – 2x – 3 = (x-1)² – 4 = 0
Solving for x, we get x=3 and x=-1.
Quadratic formula: We already know the quadratic formula.
Substituting our values, we get:
x = (2 ±√(2²-4*1*-3))/(2*1) = 3, -1
Common Mistakes to Avoid When Solving Quadratic Equations
As with any complex process, errors can occur when solving quadratic equations. We need to be aware of common mistakes and strategies to avoid them. Some common mistakes when solving quadratic equations include:
- Forgetting to multiply coefficients or variables
- Forgetting to apply the distributive property
- Forgetting to simplify
- Not checking solutions by substituting them into the original equation
Avoid these mistakes by double-checking work, simplifying wherever possible, and verifying solutions.
Real-Life Applications: How Quadratic Equations Are Used in Science and Engineering
Quadratic equations are not just abstract concepts used to solve problems in a classroom; they are used in many industries and real-word applications. For example, quadratic equations are used in computer graphics to create 3D models of objects. The height of a projectile over time can be modeled using quadratic equations, which are used in sports and civil engineering projects. The design of rollercoasters also involves complex quadratic equations.
Understanding quadratic equations is essential for success in many fields, and proficiency in them opens up opportunities for many exciting careers.
Solving Quadratic Equations Made Simple: Tips and Tricks for Success
Here are some additional tips and tricks to help you solve quadratic equations more efficiently and effectively:
- Keep a checklist of all steps for solving quadratic equations
- Practice solving problems at varying levels of difficulty
- Use small numbers to check your solutions
- Break the quadratic equation down into smaller parts to make solving easier
Conclusion
Solving quadratic equations is a complex process that requires a lot of practice and patience. By mastering the basics, avoiding common mistakes, and applying tips and tricks, we can become proficient in quadratic equation-solving. Quadratic equations are not just abstract concepts, and proficiency in them is essential for success in many fields.